CASH v. DAU
DNA testing - Cashel with the Baronets branch.
For background see
DNA testing for the Blennerhassett problem.
This page is about the tests done to prove or disprove the
theory of our descent from the Blennerhassett Baronets branch
through a lost daughter.
On the very first sample (BART.1)
the results said the Baronets theory is true.
Our family is closely related to the Blennerhassett Baronets branch.
Groups
We compare individuals from the
groups defined here.
This page will compare:
We start by considering total segment matches, using minimum segment size = 9 cM.
9 cM is generally considered significant.
We compare our family
with the Blennerhassett Baronets family.
- This looks really good.
- We have done
one to one comparisons.
- We have two great matches with the BART branch,
which is where our theory predicts we connect.
- We have two great matches with ROB.1, who descends from both ROB and YIELD.
- We have one great match with BUTLER,
which descends from YIELD.
- We have a number of other supporting matches
with the Blennerhassett Baronets branch
and the Yielding family.
- The results strongly support the Baronets theory.
We clearly descend from one or both of the two Blennerhassett-Yielding marriages
in that family.
-
Compare it with the
equivalent table for the Letitia theory (which is almost empty).
- In fact, the results are even better than they look here, because
we have
more matches on Ancestry not shown here.
They are not shown here because they are not on Gedmatch.
Is the BART.1 match evidence for the Letitia theory?
- BART.1 descends from
Arthur Blennerhassett of Blennerville
and his wife Helena Jane Mullins.
So this sample is also part of the MUL group
in our
DNA testing of the Letitia Blennerhassett theory.
-
So why not count this as evidence towards the Letitia theory?
-
Because it makes no sense to have a strong match in MUL and no match in REV or ED.
We think the match to our family is through Arthur,
not through his wife.
- Also, of course, we have the BART.6 match, which is nothing to do with MUL.
- We actually have to remove some BUTLER matches with CASH.1 here.
- BUTLER.3 and CASH.1 have a 12.4 cM match.
- BUTLER.1 (nephew of BUTLER.3) and CASH.1 have an 8.5 cM match.
- So why are they omitted?
Because through
Triangulation,
we discovered they are related to CASH.1 through a non-Cashel line
(the mother of CASH.1)
and the matches are through that line.
So we exclude them.
Looking at them is misleading.
-
This does not affect any other CASH since none are related to the mother of CASH.1.
So we can leave the others in.
- To spell it out, any match of BUTLER to those other CASH
cannot be explained by the line of the mother of CASH.1,
since none of those CASH are related to the mother of CASH.1.
So any match needs another explanation.
- This amazing false positive shows that you can get a 12.4 cM match by chance, which is shocking.
- It also shows that a DNA match is not enough.
You need to triangulate to find out which line the match is through.
The following 15 cM match between our family and the Baronets family
basically proved
that the Baronets theory is true.
This match proved the Blennerhassett link
on 26 May 2020, after 35 years of trying.
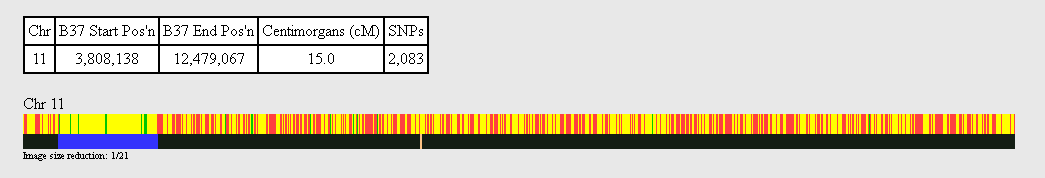
The match is between these two people:
The second great match, Oct 2020
On 18 Oct 2020, we got an even better match with the Baronets family, of 21 cM.
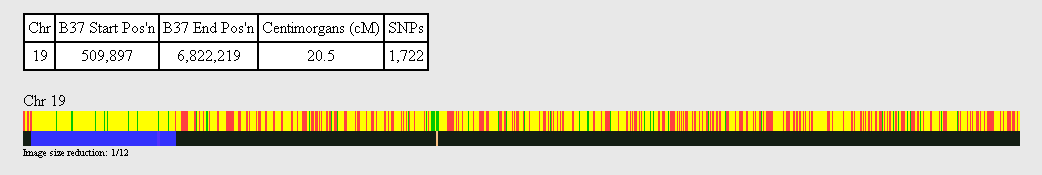
The match is between these two people:
The third great match, May 2025
On 11 May 2025, we got an even better match, with the Yielding family, of 24 cM.
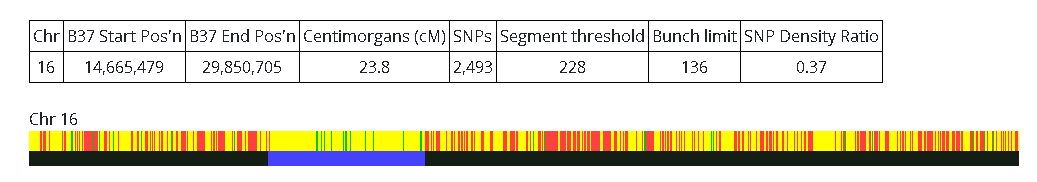
The match is between these two people:
Let us try (as some sites do)
reducing the minimum segment size and looking at total segment matches.
Here is total segment matches using minimum segment size = 7 cM.
- With this metric, the Baronets theory continues to look good.
-
Compare with the
7 cM table for the Letitia theory, which remains much weaker.
We now take a wider look at the data by showing for every match, what is the largest segment.
Largest single segment in match, in cM.
Small segments can happen by chance. Large segments much less so.
If there is a segment over 5 cM,
Gedmatch
estimates the number of generations to Most Recent Common Ancestor (MRCA).
This is an estimate from the DNA, not from the family tree.
Gedmatch can give different estimates depending on the minimum segment length you pick.
We use the closest estimate, which is the estimate given under minimum segment 5 cM.
- BART seem proven relations of our family,
with matches across different branches of our family.
- ROB.1 seems a proven relation of our family.
- BUTLER seem proven relations of our family,
with matches across different branches of our family.
-
Compare this with the
MRCA table for the Letitia theory, which is dramatically worse.
Now we consider, under the Baronets theory, the
postulated
cousin relations of CASH with DAU.
How do we estimate the cousin numbers?
This is difficult.
We take the most likely theory, which is that we descend from the 1st Baronet.
We assume
George Cashel
is somehow a grandson of the 1st Baronet.
But depending exactly how he is a grandson, these cousin numbers could vary.
e.g. If George Cashel is the natural son of the 2nd Baronet, then
we have 3.1 and 4.0 cousinships with the 7th Baronet.
If George Cashel is son of the 2nd Baronet's sibling,
those become 4.1 and 5.0 cousinships.
Until we find out exactly how George Cashel descends, we will use the bigger numbers.
- The CASH people that are closest to the origin of the Cashel family are the ones for which we can get:
- Any version of 4th cousin.
- 5.0 cousin.
- These ones are all in the group CASH 3 to CASH 9 (as laid out here, not numerically).
Here are the Gedmatch estimated MRCAs:
The pattern looks different to the postulated cousins.
None of the 4th cousins match.
It does not at first look great.
But in fact, looked at a different way, the DNA is actually a pretty good fit with the postulated cousins:
- Consider the BART group.
For each CASH sample, the closest postulated cousins are BART 6 and 7 and 1.
And indeed the best DNA matches are found in BART 6 and 1.
- Consider the BUTLER group.
For each CASH sample, the closest postulated cousin is BUTLER.3.
And indeed BUTLER.3 is where more of the best DNA matches are found.
- Consider the group CASH.3 to CASH.9 (as laid out here, not numerically).
This is where we have the closest postulated cousins to Blennerhassett
(not all of them, but most of CASH.3 to CASH.9).
And indeed it is where the best DNA matches are found.
Here are the largest segments:
Let us write out the entire list of results for
real cousins
versus the same list for
postulated cousins,
separate into lists for each type of cousin,
and sort the lists,
to see if the postulated has the same pattern as the real.
Here are the lists, for each type of cousin,
of largest segments
for real cousins
versus the same list for
postulated cousins (for CASH crossed with DAU).
We start at "4.0" cousins (there are no closer living postulated cousins).
- This looks bad.
- But we have very few samples.
- First, we have no 4.0 samples at all. Nor will we ever get any.
- We do have some 4.1 samples.
And these look really bad. How can this be?
- The 4.1 samples relate entirely to two people on the Cashel side, who are siblings.
So there is a bottleneck.
The siblings' parent might simply have not inherited much DNA in common with the small number of
Blennerhassett people we have samples of.
- We cannot get any more 4.1 samples on the Cashel side,
but we might get some more on the Blennerhassett side.
Maybe with more samples, we will finally get a decent 4.1 match.
- It is the 5th cousins comparison that makes the Baronets theory look true.
- The results are a little weaker than the known, but not by much.
The general pattern looks good.
These really are 5th cousins, or at most 6th cousins.
The Baronets theory looks true.
- The results are compatible with these people really being 6th cousins.
- The conclusion is the Baronets theory looks true.
-
We seem to be closely related to Blennerhassett, Yielding and Butler.
- Note that the finding is actually stronger than appears here,
because we have other matches on Ancestry which are not visible here.
They are not visible here because they are not on Gedmatch.
-
And we also have some
triangulation
on Gedmatch
indicating which exact lines the DNA matches are through.


